Conditions Under Which a
Constant Velocity is Observed
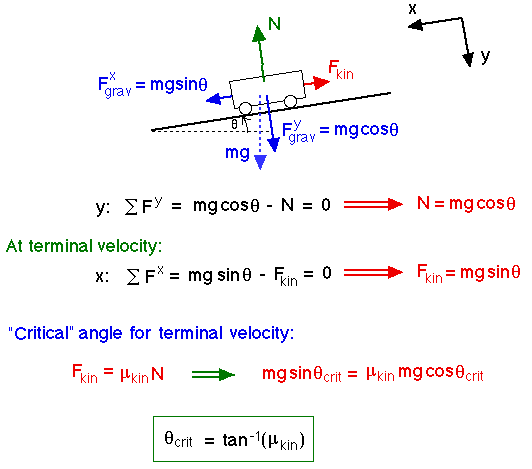
The cart maintains a constant velocity in the direction of
travel (x-direction) when the force of kinetic friction, Fkin,
balances the x-component of the gravitational force, mgsinØ, i.e.,
when Fkin = mgsinØ. By writing the force of kinetic
friction in terms of the inclination angle, Fkin =
µkin N = µkin mgcosØ, the condition
for the "critical" inclination angle at which the cart achieves a constant
velocity can be determined: mgsinØcrit =
µkin N = µkin mgcosØcrit
(Lectures 7 and
8).
Different "Regimes"
of a Cart Sliding Down an Incline
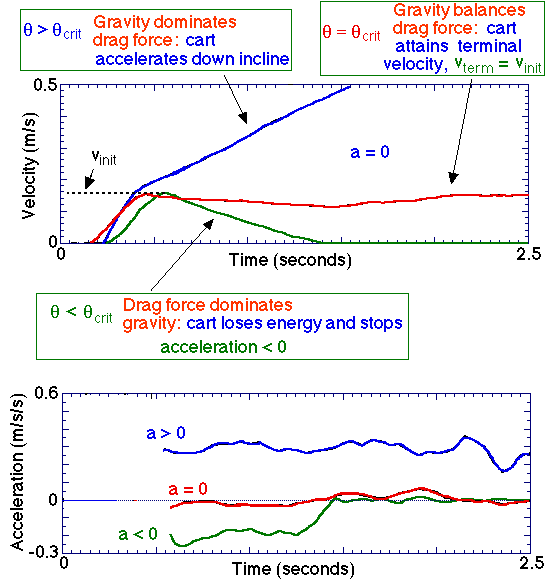
In the presence of a frictional force, a cart sliding down
an incline exhibits several different types of behavior as a function of
inclination angle. For inclination angles greater than the "critical"
angle, Ø > Øcrit =
tan-1(µkin), the gravitational force exceeds
the drag force, i.e., mgsinØ > Fkin, and the cart
accelerates down the incline. For inclination angles smaller than the
"critical" angle, Ø < Øcrit, the drag force exceeds
the gravitational force, Fkin > mgsinØ, and the cart
slows from its initial velocity, vinit, until stopping, as
energy is lost to heat due to friction. At the "critical" inclination
angle, Ø = Øcrit, the gravitational force is balanced
by the drag force, mgsinØ = Fkin, and the cart maintains
a constant velocity equal to the initial velocity of the cart
(Lectures Lecture 7 and
Lecture 8).
Back to Lab #3 Page